Question

Suppose that f(0) = −5 and f '(x) ≤ 7 for all values of x. How large can f(5) possibly be?

Answer 1

f(5) cannot exceed 30.

Explanation:

f is differentiable in all R, so we can apply the Mean Value Theorem on the interval [0,5], since f is continuous on [0,5] and differentiable on (0,5).

By the MVT, there exists some number c∈(0,5) such that
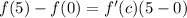`f(5)-f(0)=f'(c)(5-0)`. Substituting the value of f(0), this last equation is equivalent to
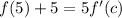`f(5)+5=5f'(c)`. But f'(x)≤7 for all values of x, in particular for x=c, so applying this bound we obtain that
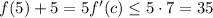`f(5)+5=5f'(c)\leq5\cdot 7=35`. Then
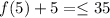`f(5)+5=\leq35`, so substracting 5 from both sides we conclude that
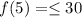`f(5)=\leq30`.