Question

The table of values represents a function ​f(x).

How much greater is the average rate of change over the interval [9, 10] than the interval [5, 8] ?

Enter your answer in the box. x


The table of values represents a function ​f(x).


How much greater is the average rate of change over the interval [9, 10] than the interval [5, 8] ?

x f(x)

5 75

6 202

7 549

8 1491

9 4052

10 11,014





Enter your answer

Answer 1

The average rate of change over the interval [9,10] is greater than that over the interval [5,8] by 6490.

Explanation:

The value of f(x) = 4052 for x = 9 and f(x) = 11014 for x = 10.

Therefore, the average rate of change of f(x) with respect to x over the interval [9,10] is given by

=
`\frac{\textrm {Total change in value of f(x)}}{\textrm {Total change in value of x}}`

=
`(11014 - 4052)/(10 - 9) = 6962`

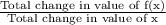
Now, the value of f(x) = 75 for x = 5 and f(x) = 1491 for x = 8.

Therefore, the average rate of change of f(x) with respect to x over the interval [5,8] is given by

=
`\frac{\textrm {Total change in value of f(x)}}{\textrm {Total change in value of x}}`

=
`(1491 - 75)/(8 - 5) = 472`

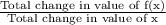
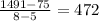
Therefore, the average rate of change over the interval [9,10] is greater than that over the interval [5,8] by (6962 - 472) = 6490. (Answer)