Question

Drag numbers to complete a function in terms of x and y for the line that contains the points (2, 4) and (6, 16). Numbers may be used once, more than once, or not at all.

Answer 1

Answer:
`y=3x-2`

Explanation:

Since there is not image attached, below is the explanation to find the equation of the line that contains the points (2, 4) and (6, 16), in Slope-Intercept form:

The Slope-Intercept form of the equation of the line is:

`y=mx+b`

Where "m" is the slope and "b" is the y-intercept.

You can find the slope of the line with this formula:

`m=(y_2-y_1)/(x_2-x_1)`

Now, given the points (2, 4) and (6, 16), you can say that:

`y_2=16\\y_1=4\\\\x_2=6\\x_1=2`

So, substituting values, you get:

`m=(16-4)/(6-2)=3`

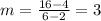
Now you can ubstitute the slope and the coordinates of one of the given points into
`y=mx+b` and solve for "b":

`4=3(2)+b\\\\4-6=b\\\\b=-2`

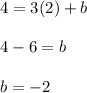
Therefore, you can determine that the equation of this line is:

`y=3x-2`