Question

a line goes through the point(6,-2)and has a slope of -3 what is the value of a if the point (a,7) lies on the line​

Answer 1

a= 3

Explanation:

:)

Answer 2

The value of a is "3", to complete the coordinate point (3,7) on the line.

Explanation:

We are given the slope of the line, and also one point through which the line passes. Therefore, we can find the actual equation of the line by using the slope-point form of the line:

`y-y_0=m(x-x_0)\\y-(-2)=(-3)(x-6)\\y+2=-3x+18\\y=-3x+18-2\\y=-3x+16`

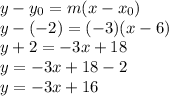
Now, we need to find which value of "x" renders a y-value of "7", to complete the coordinate pair (a, 7) as requested. Therefore, we ask for the y-value in the previous equation to be "7", and solve for its associated x-value:

`y=-3x+16\\7=-3x+16\\7-16=-3x\\-9=-3x\\x=(-9)/(-3) \\x=3`

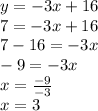
Therefore, x = 3