Question

At the gas station the equation c=2.80g gives the relationship .....

Answer 1

`\$0.52\ per\ gallon`

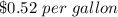
Explanation:

Let

g ----> number of gallons of gasoline

c ---> the total cost in dollars

we have

First gas station

`c=2.80g`

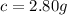
This is a proportional relationship between the variables c and g

The constant of proportionality k or unit rate (slope) is equal to

`k=\$2.80\ per\ gallon`

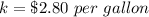
Second gas station

we have the ordered pairs

(2.5,8.30) and (5,16.60)

This is a proportional relationship between the variables c and g

The constant of proportionality k is equal to

`k=(16.6)/(5)=\$3.32\ per\ gallon`

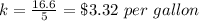
the linear equation is

`c=3.32g`

To find out how much per gallon would Billy save, subtract the unit rate of the Gas station 1 from the unit rate of the Gas station 2

so

`\$3.32\ per\ gallon-\$2.80\ per\ gallon=\$0.52\ per\ gallon`