Question

Consider a golf club hitting a golf ball that results in the following graph of force versus time on a 45 gram golf ball. If the final velocity of the ball has a magnitude of 41 m/s, determine the value of Fmax

Answer 1

The question is missing the graph. So, the graph is attached below.

Answer:

The value of
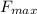`F_(max)` is 7380 N.

Step-by-step explanation:

Given:

Mass of the ball is,
`m=45\ g=0.045\ kg`

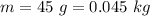
Initial velocity of the ball is,
`u=0\ m/s(Assuming)`

Final velocity of the ball is,
`v=41\ m/s`

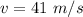
From the graph,

Time interval for which the force acts,
`\Delta t=0.5\ ms=0.5* 10^(-3)\ s`

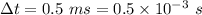
Height of the triangle is equal to the maximum force acting on the ball =
`F_(max)`

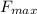
Now, we know that, impulse acting on the ball is equal to the area under the curve of force and time graph.

So, impulse is equal to the area of the triangle and is given as:

Impulse =
`(1)/(2)* base* height`

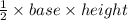
Here, base is time interval
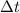`\Delta t` and height is
`F_(max)`.

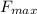Impulse =
`(1)/(2)* (0.5* 10^(-3))* F_(max)`

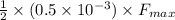
Impulse =
`0.25* 10^(-3)F_(max)`

Now, we also know that, impulse is equal to the change in momentum of a body.

Therefore, change in momentum (Δp) is given as:

`\Delta p=m(v-u)\\\Delta p = 0.045(41-0)=1.845\ kgms^(-1)`

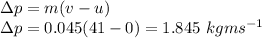
Now, change in momentum is equal to impulse acting on the ball. Thus,

`0.25* 10^(-3)F_(max)=1.845\\\\F_(max)=(1.845)/(0.25* 10^(-3))\\\\F_(max)=7380\ N`

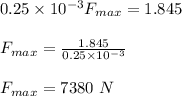
Therefore, the value of
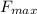`F_(max)` is 7380 N.