We have
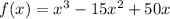 .
.
To find zeros of this function we equate it with 0
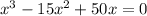 .
.
First we factor out x
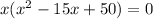
where we find that first zero is
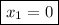 .
.
Then we look at the expression in parentheses
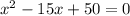
using Viéts rule (factorisation)
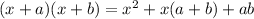
we can rewrite the equality
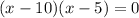 .
.
If either of the terms is zero then the equality is true so we get two more zeros
 and
and
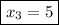 .
.
Hope this helps.