Answer:
We conclude that at a 5% significance level that the mean delivery time is longer than what the pizza shop claims.
Explanation:
We are given the following in the question:
Population mean, μ = 30 minutes
Sample mean,
 = 31.5 minutes
= 31.5 minutes
Sample size, n = 41
Alpha, α = 0.05
Sample standard deviation, s = 3.5 minutes
First, we design the null and the alternate hypothesis

We use one-tailed z test to perform this hypothesis.
Formula:
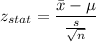
Putting all the values, we have

Now,
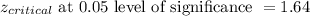
Since,
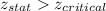
We reject the null hypothesis and accept the alternate hypothesis.
Thus, we conclude that at a 5% significance level that the mean delivery time is longer than what the pizza shop claims.