Question

Which classification describes the following syastem of equations? x=5 y=6 -x - y +z = 0

Answer 1

Is a consistent independent system

Explanation:

we know that

If a system has at least one solution, it is said to be consistent .

If a consistent system has exactly one solution, it is independent .

If a consistent system has an infinite number of solutions, it is dependent

If a system not have solution, it is said to be inconsistent

we have

`x=5` ----> equation A

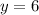`y=6` ----> equation B

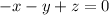`-x-y+z=0` ----> equation C

substitute equation A and equation B in equation C

`-5-6+z=0`

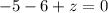
Solve for z

`z=11`

The solution is the point (5,6,11)

The system has only one solution

therefore

Is a consistent independent system