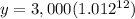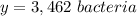Question

A culture started with 3,000 bacteria. After 8 hours, it grew to 3,300 bacteria. Predict how many bacteria will be present after 12 hours. Round your answer to the nearest whole number.

Answer 1

`3,462\ bacteria`

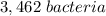
Explanation:

In this problem we have a exponential function of the form

`y=a(b^x)`

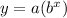
where

x is the time in hours

y is the numbers of bacteria

a is the initial value

b is the base

r is the rate of growth

b=(1+r)

we have that

`a=3,000\ bacteria`

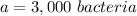
For x=8, y=3,300

substitute in the exponential function

`3,300=3,000(b^8)`

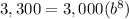
solve for b

`1.1=(b^8)`

`b=\sqrt[8]{1.1}`

`b=1.0120`

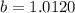
Find the value of r

`r=b-1=1.0120-1=0.0120=1.20\%`

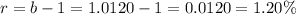
The equation is equal to

`y=3,000(1.012^x)`

For x=12 hours

substitute the value of x in the equation

`y=3,000(1.012^(12))`

`y=3,462\ bacteria`