Answer:
The claim is having statistical evidence.
Explanation:
Given that faculty members at a large university are irritated by students’ cell phones. The faculty has found over the past several semesters that a cell phone rings in class on average 20 times per semester.
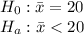
(left tailed test at 5% confidence)
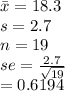
Mean difference = -1.7
Test statitstic t = mean diff/std error = -2.744
df =18
p value = 0.006671
Since p < 0.05 we reject H0
The claim is having statistical evidence.