Question

Conduct the following test at the alpha equals0.05 level of significance by determining​

(a) the null and alternative​ hypotheses,
(b) the test​ statistic, and​
(c) the critical value.

Assume that the samples were obtained independently using simple random sampling. Test whether p 1 not equals p 2 . Sample data are x 1 equals 28 ​, n 1 equals 255 ​, x 2 equals 36 and n 2 equals 302 .

​(a) Choose the correct null and alternative hypotheses below.

A. Upper H 0 : p 1 equals p 2 versus Upper H 1 : p 1 greater than p 2 Your answer is correct.
B. Upper H 0 : p 1 equals 0 versus Upper H 1 : p 1 not equals 0
C. Upper H 0 : p 1 equals p 2 versus Upper H 1 : p 1 not equals p 2
D. Upper H 0 : p 1 equals p 2 versus Upper H 1 : p 1 less than p 2 ​
(b) Determine the test statistic. z0equals nothing ​(Round to two decimal places as​ needed.)

Answer 1

z=0.6074

Explanation:

Given that we have to Conduct the following test at the alpha equals0.05 level of significance by determining​

Test whether p 1 not equals p 2 .

Suitable hypotheses would be

B. Upper H 0 : p 1 equals 0 versus Upper H 1 : p 1 not equals 0

(Two tailed test at 5% level)

Group I II total

n 255 305 557

x 28 36 64

p 0.1098 0.1180 0.1149

p difference = -0.0082

Std error for difference =
`\sqrt{(0.1149*0.8851)/(557) } \\=0.0135`

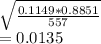
Test statistic Z = p diff/std error

= 0.6074

p =0.2718

Since p >alpha we accept H0

z=0.6074