Question

An inlet pipe can fill an artificial pond in 70 min. An outlet pipe can empty the same pond in 200 min. With both pipes open, how long will it take to fill the pond?

Answer 1

It takes 107.692 minutes approximately to fill the pond

Solution:

Given that inlet pipe can fill an artificial pond in 70 min

So the inlet pipe can fill
`(1)/(70)` of the pond in 1 min

An outlet pipe can empty the same pond in 200 min

So the outlet pipe can empty
`(-1)/(200)` of pond in 1 min

Here negative sign denotes "empties the pond"

To find: With both pipe open, time taken to fill the pond

Let "x" be the time taken by pipes to fill the pond

With both pipes are left on, so together their rate is:

`(1)/(x) = (1)/(70) - (1)/(200)`

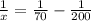
On solving,

`(1)/(x) = (200 - 70)/(14000)\\\\(1)/(x) = (130)/(14000)\\\\x = (14000)/(130) = 107.692`

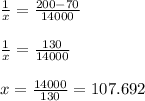
Thus it takes 107.692 minutes approximately to fill the pond