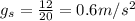Answer:
gs = 0.6 m/s^2
Step-by-step explanation:
Given data:
velocity = 12 m/s
height s = 12t -(1/2) g_s t^2
Given velocity is the derivatives of height
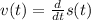
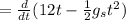
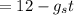
when velocity tend to 0 , maximum height is reached
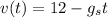


at t = 20 sec ball reached the max height, so