Answer:
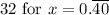
Explanation:
It looks like you want the sum of digits of the product ...
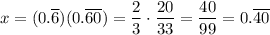
Since the decimal fraction starts off with 4 and has an even number of repeating digits, there will be 8 4's and 7 0's in the sum of the first 15 decimal digits:
sum = 8×4 +7×0 = 32
The sum of the first 15 digits after the decimal point is 32.