Question

A rectangle has a side that is 16 feet and another side there's 1/2 that links a square has a perimeter of 48 feet how much greater is the area of the Square in the area of the rectangle

Answer 1

Area of rectangle = 128 square feet

Explanation:

Given:- A rectangle with side(a)=16 feet, side (b) =
`(1)/(2)` that links to a square.

perimeter (p) = 48 feet.

To find:- area of the square of the rectangle=?

Now,

`Perimeter\ of\ square\ (p) = (2* a)+(2* b)`

`48=(2* 16)+(2* b)`

`48=32+2b`

`2b=48-32`

`2b=16`

`b=(16)/(2)`

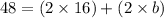
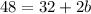
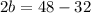
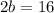
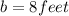`b=8 feet` -------(equation 1)

(8 is half of square of 4=16,
`4^(2)=16,\ (16)/(2) = 8`)

Now, to find the area of square:-

Area of square (A) = Length
`*` breadth

Area of square (A)= side a
`*` side b

A= 16
`*` 8

`\therefore`A = 128 square feet

Therefore Area of rectangle = 128 square feet