Answer:
The value of the magnetic field is 2.01 T when Hall voltage is 1.735 mV
Step-by-step explanation:
The frequency of the cyclotron can help us find the magnitude of the magnetic field, thus then we can compare the effect of increasing Hall voltage on the probe.
Magnetic field magnitude at initial Hall voltage.
The cyclotron frequency can be written in terms of the magnetic field magnitude as follows
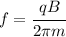
Solving for the magnetic field.
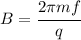
Thus we can replace the given information but in Standard units, also remembering that the mass of a proton is
 and its charge is
and its charge is
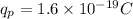 .
.
So we get
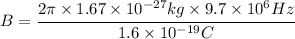
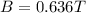
We have found the initial magnetic field magnitude of 0.636 T
Magnetic field magnitude at increased Hall voltage.
The relation given by Hall voltage with the magnetic field is:
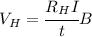
Thus if we keep the same current we can write for both cases:
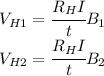
Thus we can divide the equations by each other to get
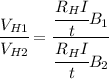
Simplifying
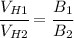
And we can solve for
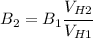
Replacing the given information we get

We get
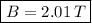
Thus when the Hall voltage is 1.735 mV the magnetic field magnitude is 2.01 T