Question

A marketing research consultant hired by Coca-Cola is interested in determining if the proportion of customers who prefer Coke to other brands is over 50%.

A random sample of 200 consumers was selected from the market under investigation, 55% favored Coca-Cola over other brands. Additional information is presented below.Sample proportion0.55Standard error of sample proportion0.03518Z test statistic1.4213p-value0.07761 1- If you were to conduct a hypothesis test to determine if greater than 50% of customers prefer Coca-Cola to other brands, would you conduct a one-tail or a two-tail hypothesis test? Explain your answer.2- How many customers out of the 200 sampled must have favored Coke in this case?3- Using a 5% significance level, can the marketing consultant conclude that the proportion of customers who prefer Coca-Cola exceeds 50%? Explain your answer.4- If you were to use a 1% significance level, would the conclusion from part c change? Explain your answer.

Answer 1

1) test is one tail hypothesis test.

2) 110 sampled customers must have favored Coke.

3) at 5% significance, We cannot conclude that the proportion of customers who prefer Coca-Cola exceeds 50%.

4) at 1% significance level, the conclusion would not change.

Explanation:

1) Let p be the proportion of customers who prefer Coke to other brands

• 
  `H_(0)`: p=0.50

• 
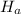  `H_(a)`: p>0.50

Since the alternative hypothesis claims p more than 0.50, this test is one tail hypothesis test.

2) Out of a random sample of 200 consumers, 55% favored Coca-Cola over other brands. Thus 200 × 0.55 = 110 sampled customers must have favored Coke.

3) at 5% significance level, p-value =0.07761 >0.05, therefore we fail to reject the null hypothesis. We cannot conclude that the proportion of customers who prefer Coca-Cola exceeds 50%.

4) at 1% significance level, p-value =0.07761 >0.01, thus the conclusion does not change