Question

Assume the distribution of commute times to a major city follows the normal probability distribution and the standard deviation is 4.5 minutes. A random sample of 38 commute times is given below in minutes. Use Excel to find the 98% confidence interval for the mean travel time in minutes. Round your answers to one decimal place and use ascending order. Time 21 15 35 40 40 34 32 11 18 35 9 31 15 29 41 37 40 10 31 14 39 14 11 33 38 21 35 33 34 27 31 26 35 27 32 18 40 17

Answer 1

(25.9, 29.3)

Explanation:

A 98% confidence interval for μ is (x¯−zα/2σn−−√,x¯+zα/2σn−−√). Here, α=0.02, σ=4.5, and n=38. Use Excel to calculate the 98% confidence interval.1. Open Excel, enter the given data in column A, and find the sample mean, x¯, using the AVERAGE function. Thus, the sample mean, rounded to one decimal place, is x¯=27.6.2. Click on any empty cell, enter =CONFIDENCE.NORM(0.02,4.5,38), and press ENTER.3. The margin of error, rounded to one decimal place, is zα/2σn−−√≈1.7. The confidence interval for the population mean has a lower limit of 27.6−1.7=25.9 and an upper limit of 27.6+1.7=29.3.Thus, the 98% confidence interval for μ is (25.9, 29.3).

Answer 2

The 98% confidence interval would be given by (25.904;29.306)

Explanation:

1) Previous concepts

A confidence interval is "a range of values that’s likely to include a population value with a certain degree of confidence. It is often expressed a % whereby a population means lies between an upper and lower interval".

The margin of error is the range of values below and above the sample statistic in a confidence interval.

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

The data is:

21, 15, 35, 40 ,40 ,34, 32, 11, 18, 35, 9, 31, 15, 29, 41, 37, 40, 10, 31, 14, 39, 14, 11, 33, 38, 21, 35, 33, 34, 27, 31, 26, 35, 27, 32, 18, 40, 17

2) Compute the sample mean and sample standard deviation.

In order to calculate the mean and the sample deviation we need to have on mind the following formulas:

`\bar X= \sum_(i=1)^n (x_i)/(n)`

`s=\sqrt{(\sum_(i=1)^n (x_i-\bar X))/(n-1)}`

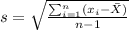
=AVERAGE(21, 15, 35, 40 ,40 ,34, 32, 11, 18, 35, 9, 31, 15, 29, 41, 37, 40, 10, 31, 14, 39, 14, 11, 33, 38, 21, 35, 33, 34, 27, 31, 26, 35, 27, 32, 18, 40, 17)

On this case the average is
`\bar X= 27.605`

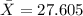
=STDEV.S(21, 15, 35, 40 ,40 ,34, 32, 11, 18, 35, 9, 31, 15, 29, 41, 37, 40, 10, 31, 14, 39, 14, 11, 33, 38, 21, 35, 33, 34, 27, 31, 26, 35, 27, 32, 18, 40, 17)

The sample standard deviation obtained was s=10.184

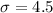`\sigma=4.5` represent the population standard deviation

3) Find the critical value t* Use the formula for a CI to find upper and lower endpoints

In order to find the critical value we need to take in count that our sample size n =38 >30 and on this case we know about the population standard deviation, so on this case we need to use the z distribution. Since our interval is at 98% of confidence, our significance level would be given by
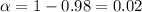`\alpha=1-0.98=0.02` and
`\alpha/2 =0.01`.

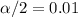We can find the critical values in excel using the following formulas:

"=NORM.INV(0.01,0,1)" for
`z_(\alpha/2)=-2.33`

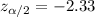
"=T.INV(1-0.01,0,1)" for
`z_(1-\alpha/2)=2.33`

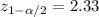
The confidence interval for the mean is given by the following formula:

`\bar X \pm z_(\alpha/2)(\sigma)/(√(n))`

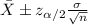
And we can use Excel to calculate the limits for the interval

Lower interval : "=27.605 -2.33*(4.5/SQRT(38))" =25.904

Upper interval : "=27.605 +2.33*(4.5/SQRT(38))" =29.306

So the 98% confidence interval would be given by (25.904;29.306)