The number of bags of chips sold is 8 and the number of packs of cookies sold is 12
Solution:
Let "a" be the number of bags of chips sold
Let "b" be the number of packs of cookies sold
Given that,
Cost of one bag of chips = $ 1.50
Cost of one pack of cookies = $ 1.25
Yesterday Abigail made $27 from selling a total of 20 bags of chips and packs of cookies
So we can frame a equation as:
Number of bags of chips sold + number of packs of cookies sold = 20
a + b = 20 ---- eqn 1
Also given that Abigail made $27 from selling
number of bags of chips sold x Cost of one bag of chips + number of packs of cookies sold x Cost of one pack of cookies = $ 27
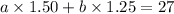
1.5a + 1.25b = 27 ---- eqn 2
Let us solve eqn 1 and eqn 2 to find values of "a" and "b"
From eqn 1,
a = 20 - b ---- eqn 3
Substitute eqn 3 in eqn 2
1.5(20 - b) + 1.25b = 27
30 - 1.5b + 1.25b = 27
-0.25b = -3
b = 12
Therefore from eqn 3,
a = 20 - b = 20 - 12 = 8
a = 8
Thus we have:
number of bags of chips sold = 8
number of packs of cookies sold = 12