Answer:
see explanation
Explanation:
The equation of a line in point- slope form is
y - b = m(x - a)
where m is the slope and (a, b) a point on the line
y - 3 = 4(x + 2) ← is in point- slope form
with slope m = 4
Given a line with slope m then the slope of a line perpendicular to it is
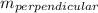 = -
= -
 = -
= -

and (a, b) = ( - 2, 6), then
y - 6 = -
 (x - )- 2)), that is
(x - )- 2)), that is
y - 6 = -
 (x + 2)
(x + 2)