Answer:
58.9% produced produced peppers weighing between 13 and 16 pounds.
Explanation:
We are given the following information in the question:
Mean, μ = 15
Standard Deviation, σ = 1.75
We are given that the distribution of weight of peppers is a bell shaped distribution that is a normal distribution.
Formula:
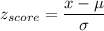
P(peppers weighing between 13 and 16 pounds)
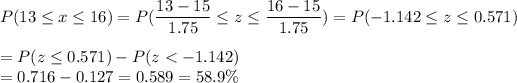
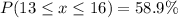
58.9% produced produced peppers weighing between 13 and 16 pounds.