Question

An escalator lifts people to the second floor of a building, 20 ft

above the first floor. The escalator rises at a 30° angle. To the
nearest foot, how far does a person travel from the bottom to
the top of the escalator?

Answer 1

The person has to travel 40 feet from the bottom to the top of the escalator

Solution:

Given that escalator lifts people to the second floor of a building, 20 ft

above the first floor

The escalator rises at a 30° angle

To find: Distance person travel from the bottom to the top of the escalator

The above scenario forms a right angled triangle where the escalator follows the path of the hypotenuse.

The diagram is attached below

In the right angled triangle ABC,

AC represents Distance person travel from the bottom to the top of the escalator

AB = 20 feet

angle ACB = 30 degree

We know that,

`sin \theta = (opposite)/(hypotenuse)`

`sin 30 = (20)/(AC)`

`(1)/(2) = (20)/(AC)\\\\AC = 20 * 2 = 40`

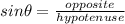
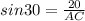
So the person has to travel 40 feet from the bottom to the top of the escalator