Explanation:
Given precision is a standard deviation of s=1.8, n=12, target precision is a standard deviation of σ=1.2
The test hypothesis is
H_o:σ <=1.2
Ha:σ > 1.2
The test statistic is
chi square =
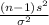
=
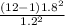
=24.75
Given a=0.01, the critical value is chi square(with a=0.01, d_f=n-1=11)= 3.05 (check chi square table)
Since 24.75 > 3.05, we reject H_o.
So, we can conclude that her standard deviation is greater than the target.