Question

At what temperature would a given reaction become spontaneous if H = ______ kJ and S = ______ J/K? The Instructor will choose a value for delta H between 50 kJ and 250 kJ and delta S between 100 J/K and 300 J/K. The correct answer must show the relationship between delta H and delta S that is used to calculate the temperature at which the change occurs as well as the calculations themselves. The final answer must have the correct units with it. Any answer that simply shows a numerical value with units will NOT be accepted.

Answer 1

`T > (\Delta H^o)/(\Delta S^o)`

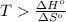
Step-by-step explanation:

The spontaneity of a reaction is described by the Gibbs free energy change. Several statements should be emphasized in the context of this problem:

• the change in Gibbs free energy relates enthalpy, entropy and temperature by the following equation:
  `\Delta G^o = \Delta H^o - T\Delta S^o`;
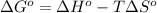• if the change in the Gibbs free energy is negative, the reaction is spontaneous, that is:
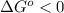  `\Delta G^o < 0` or
  `\Delta H^o - T\Delta S^o < 0`;
• if the change in the Gibbs free energy is positive, the reaction is non-spontaneous, that is:
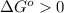  `\Delta G^o > 0` or
  `\Delta H^o - T\Delta S^o > 0`.

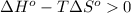In this problem, we wish to find the temperature at which the given reaction becomes spontaneous, that is, the minimum T value for which we obtain a negative value of the Gibbs free energy change:

`\Delta H^o - T\Delta S^o < 0`

Rearrange the inequality:

`\Delta H^o < T\Delta S^o`

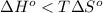
Divide both sides by the change in entropy:

`T > (\Delta H^o)/(\Delta S^o)`

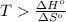
Let's take an example. Let's say that we have a change in enthalpy as 50 kJ/mol and a change in entropy of 100 J/K, then:

`T > (50\cdot 10^3 J/mol)/(100 J/K) = 500 K`

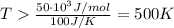
Thus, for these conditions, our reaction would become spontaneous at 500 K and above.