Question

Assume that a simple random sample has been selected from a normally distributed population and test the given claim. Identify the null and alternative​ hypotheses, test​ statistic, P-value, and state the final conclusion that addresses the original claim. In a manual on how to have a number one​ song, it is stated that a song must be no longer than 210 seconds. A simple random sample of 40 current hit songs results in a mean length of 234.1 sec and a standard deviation of 54.52 sec. Use a 0.05 significance level and the accompanying Minitab display to test the claim that the sample is from a population of songs with a mean greater than 210 sec. What do these results suggest about the advice given in the​ manual?

Answer 1

There is enough evidence to conclude that the sample is from a population of songs with a mean greater than 210 sec.

Explanation:

We are given the following in the question:

Population mean, μ = 210 seconds

Sample mean,
`\bar{x}` = 234.1 sec

Sample size, n = 40

Sample standard deviation, s = 54.52 sec

First, we design the null and the alternate hypothesis

`H_(0): \mu \leq 210\text{ seconds}\\H_A: \mu > 210\text{ seconds}`

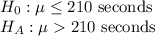
We use one-tailed t test to perform this hypothesis.

Formula:

`t_(stat) = \displaystyle\frac{\bar{x} - \mu}{(\sigma)/(√(n)) }`

Putting all the values, we have

`t_(stat) = \displaystyle(234.1 - 210)/((54.52)/(√(40)) ) = 2.795`

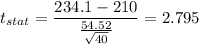
Now,
`t_(critical) \text{ at 0.05 level of significance, 9 degree of freedom } = 1.684`

We calculate the p-value with the help of standard normal table.

P-value = 0.004005

Since,

`t_(stat) > t_(critical)`

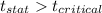
We fail to accept the null hypothesis and accept the alternate hypothesis.

Thus, there is enough evidence to conclude that the sample is from a population of songs with a mean greater than 210 sec.