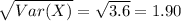Question

Twelve of the top twenty finishers in the 2009 PGA Championship at Hazeltine National Golf Club in Chaska, Minnesota, used a Titleist brand golf ball (GolfBallTest website, November 12, 2009). Suppose these results are representative of the probability that a randomly selected PGA Tour player uses a Titleist brand golf ball. For a sample of 15 PGA Tour players, make the following calculations.

Round your answers to four decimal places, if necessary.

a. Compute the probability that exactly 10 of the 15 PGA Tour players use a Titleist brand golf ball.
b. Compute the probability that more than 10 of the 15 PGA Tour players use a Titleist brand golf ball.
c. For a sample of 15 PGA Tour players, compute the expected number of players who use a Titleist brand golf ball.
d. For a sample of 15 PGA Tour players, compute the variance and standard deviation of the number of players who use a Titleist brand golf ball.

i. Variance
ii. Standard deviation

Answer 1

a) 18.59% probability that exactly 10 of the 15 PGA Tour players use a Titleist brand golf ball.

b) 21.73% probability that more than 10 of the 15 PGA Tour players use a Titleist brand golf ball.

c) The expected number of players who use a Titleist brand golf ball is 9.

d) The variance is 3.6. The standard deviation is 1.90.

Explanation:

For each player, there are only two possible outcomes. Either they use a Titleist brand golf ball, of they do not. This means that we use the binomial probability distribution to solve this problem.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

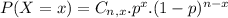
In which
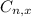`C_(n,x)` is the number of different combinatios of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

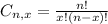
And p is the probability of X happening.

This distribution has an expected value of:

`E(X) = np`

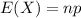
And a variance of:

`Var(X) = np(1-p)`

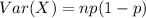
In this problem we have that:

`p = (12)/(20) = 0.6, n = 15`.

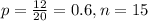a. Compute the probability that exactly 10 of the 15 PGA Tour players use a Titleist brand golf ball.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 10) = C_(15,10)*(0.6)^(10).(0.4)^(5) = 0.1859`

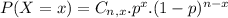
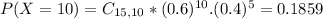
There is a 18.59% probability that exactly 10 of the 15 PGA Tour players use a Titleist brand golf ball.

b. Compute the probability that more than 10 of the 15 PGA Tour players use a Titleist brand golf ball.

This is
`P(X > 10) = P(X = 11) + P(X = 12) + P(X = 13) + P(X = 14) + P(X = 15) = 0.2173`.

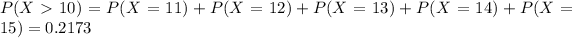There is a 21.73% probability that more than 10 of the 15 PGA Tour players use a Titleist brand golf ball.

c. For a sample of 15 PGA Tour players, compute the expected number of players who use a Titleist brand golf ball.

`E(X) = np = 15*0.6 = 9`

The expected number of players who use a Titleist brand golf ball is 9.

d. For a sample of 15 PGA Tour players, compute the variance and standard deviation of the number of players who use a Titleist brand golf ball.

`Var(X) = np(1-p) = 15*0.6*(0.4) = 3.6`

`√(Var(X)) = √(3.6) = 1.90`