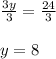Answer:
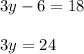
The value of "y" is:
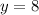
Explanation:
Given the following equation:
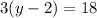
1. You must apply the Distributive property on the left side of the equation (This is:
 ). Then:
). Then:
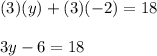
2. Now you need to apply the Addition Property of Equality. Remember that this states that:
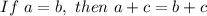
So, adding 6 to both sides of the equation, you get:
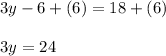
3. Finally, you can apply the Division Property of Equality, which states that:
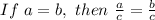
So, dividing both sides of the equation by 3, you get: