Question

What is the 36th term of the sequence 355,348,341

Answer 1

110

Explanation:

Given sequence: 355,348,341,.....

Now, finding the 36th term of the sequence.

∴ we know the formula for sequence is
`T_n= a+(n-1)d`

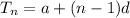
Where a is the first term, n is the nth term which we need to find and d is the difference between two adjacent term.

∴ a= 355

n= 36

d=
`348-355= -7`

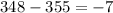
Next, substituting the value in the formula

`T_3_6= 355+(36-1)* -7`

⇒
`T_3_6= 355+(35)* -7`

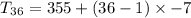
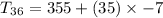
Opening the parenthesis

⇒
`T_3_6= 355+ 35* -7= 355-245`

∴
`T_3_6= 110`

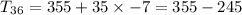
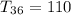
∴ 36th term of the sequence is 110.