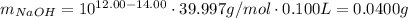Answer:
0.0400 g for the example given below.
Step-by-step explanation:
pH value is not provided, so we'll solve this problem in a general case and then we will use an example to justify it.
- By definition,
![pH = -log[H_3O^+]](https://img.qammunity.org/2020/formulas/chemistry/high-school/ced4ktntln1flx5tyce2xmc2f2fst29mie.png) .
. - NaOH is a strong base, as it's a hydroxide formed with a group 1A metal, so it dissociates fully in water by the equation:
 .
. - From the equation above, using stoichiometry we can tell that the molarity of hydroxide is equal to the molarity of NaOH:
![[NaOH] = [OH^-]](https://img.qammunity.org/2020/formulas/chemistry/high-school/esozvoza9u1wkxn4movfkb16evukvmz59i.png) .
. - Concentration of hydroxide is then equal to the ratio of moles of NaOH and the volume of the given solution. Moles themselves are equal to mass over molar mass, so we obtain:
![[OH^-] = [NaOH] = (n_(NaOH))/(V) = (m_(NaOH))/(M_(NaOH)V)](https://img.qammunity.org/2020/formulas/chemistry/high-school/ejngs4j5f09hj17vbja5b8h3j52gsi51sl.png) .
. - We also know that
![pOH = 14.00 - pH = -log[NaOH]](https://img.qammunity.org/2020/formulas/chemistry/high-school/ffe985qxjg64cgc3ubyqtdo6q7g9rpenz0.png) . Take the antilog of both sides:
. Take the antilog of both sides:
![10^(-pOH) = 10^(pH - 14.00) = [NaOH] = (m_(NaOH))/(M_(NaOH)V)](https://img.qammunity.org/2020/formulas/chemistry/high-school/jbh6n8ajcfsxoixxrpeuwnfnojdmmlbxn4.png) .
. - Solve for the mass of NaOH:
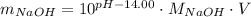 .
.
Now, let's say that pH is given as 12.00 and we use a 100-ml volumetric flask. Then we would obtain: