Question

A physical fitness association is including the mile run in its secondary-school fitness test. The time for this event for boys in secondary school is known to possess a normal distribution with a mean of 450 seconds and a standard deviation of 50 seconds. The fitness association wants to recognize the fastest 10% of the boys with certificates of recognition. What time would the boys need to beat in order to earn a certificate of recognition from the fitness association? (8 pts)

Answer 1

The boys need to complete the run in 385.9 seconds or less in order to earn a certificate of recognition from the fitness association.

Explanation:

We are given the following information in the question:

Mean, μ = 450

Standard Deviation, σ = 50

We are given that the distribution of time for fitness test is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

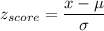
We have to find the value of x such that the probability is 0.10

P(X<x) = 0.10

`P( X < x) = P( z < \displaystyle(x - 450)/(50))= 0.10`

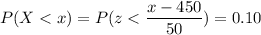
Calculation the value from standard normal z table, we have,

`P(z\leq -1.282) = 0.10`

`\displaystyle(x - 450)/(50) = -1.282\\\\x = 385.9`

Hence, boys need to complete the run in 385.9 seconds or less in order to earn a certificate of recognition from the fitness association.