Question

A mechanic sells a brand of automobile tire that has a life expectancy that is normally​ distributed, with a mean life of 31 comma 000 miles and a standard deviation of 2800 miles. He wants to give a guarantee for free replacement of tires that​ don't wear well. How should he word his guarantee if he is willing to replace approximately​ 10% of the​ tires?

Answer 1

Hence, if the tire wears in less than equal to 27410.4 miles, then the mechanic will replace approximately​ 10% of the​ tires.

Explanation:

We are given the following information in the question:

Mean, μ = 31,000

Standard Deviation, σ = 2,800

We are given that the distribution of life expectancy is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

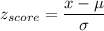
We have to find the value of x such that the probability is 0.10

P(X<x) = 0.10

`P( X < x) = P( z < \displaystyle(x - 31000)/(2800))= 0.10`

Calculation the value from standard normal z table, we have,

`P(z\leq -1.282) = 0.10`

`\displaystyle(x - 31000)/(2800) = -1.282\\\\x = 27410.4`

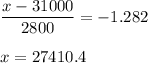
Hence, if the tire wears in less than equal to 27410.4 miles, then the mechanic will replace approximately​ 10% of the​ tires.