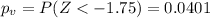Question

A practice law exam has 100 questions, each with 5 possible choices. A student took the exam and received 13 out of 100.If the student guesses the whole test, the expected number of correct answers is 20 with a standard error of .Compute the z-test statistic for the observed value 13.Find the observed significance level or P-value of the statistic.

Answer 1

`z=(13-20)/(4)=-1.75`

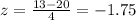
Assuming:

H0:
`\mu \geq 20`

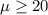
H1:
`\mu <20`

`p_v = P(Z<-1.75) = 0.0401`

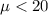
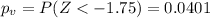
Explanation:

The binomial distribution is a "DISCRETE probability distribution that summarizes the probability that a value will take one of two independent values under a given set of parameters. The assumptions for the binomial distribution are that there is only one outcome for each trial, each trial has the same probability of success, and each trial is mutually exclusive, or independent of each other".

Let X the random variable of interest (number of correct answers in the test), on this case we now that:

`X \sim Binom(n=100, p=0.2)`

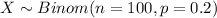
The probability mass function for the Binomial distribution is given as:

`P(X)=(nCx)(p)^x (1-p)^(n-x)`

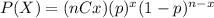
Where (nCx) means combinatory and it's given by this formula:

`nCx=(n!)/((n-x)! x!)`

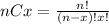
We need to check the conditions in order to use the normal approximation.

`np=100*0.2=20 \geq 10`

`n(1-p)=20*(1-0.2)=16 \geq 10`

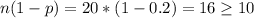
So we see that we satisfy the conditions and then we can apply the approximation.

If we appply the approximation the new mean and standard deviation are:

`E(X)=np=100*0.2=20`

`\sigma=√(np(1-p))=√(100*0.2(1-0.2))=4`

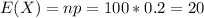
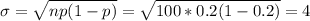
So we can approximate the random variable X like this:

`X\sim N(\mu =20, \sigma=4)`

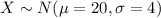
Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

The Z-score is "a numerical measurement used in statistics of a value's relationship to the mean (average) of a group of values, measured in terms of standard deviations from the mean". The letter
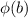`\phi(b)` is used to denote the cumulative area for a b quantile on the normal standard distribution, or in other words:
`\phi(b)=P(z<b)`

The z score is given by this formula:

`z=(x-\mu)/(\sigma)`

If we replace we got:

`z=(13-20)/(4)=-1.75`

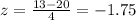
Let's assume that we conduct the following test:

H0:
`\mu \geq 20`

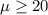
H1:
`\mu <20`

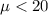
We want to check is the score for the student is significantly less than the expected value using random guessing.

So on this case since we have the statistic we can calculate the p value on this way:

`p_v = P(Z<-1.75) = 0.0401`