Question

A study was made to determine whether more Italians than Americans prefer white champagne to pink champagne at weddings. Of the 300 Italians selected at​ random, 74 preferred white​ champagne, and of the 400 Americans​ selected, 60 preferred white champagne. Can we conclude that a higher proportion of Italians than Americans prefer white champagne at​ weddings? Use a 0.05 level of significance.

Answer 1

To determine whether a higher proportion of Italians than Americans prefer white champagne at weddings, we need to conduct a hypothesis test. First, state the null and alternative hypotheses. Then, calculate the test statistic and compare it to the critical value at a significance level of 0.05.

Step-by-step explanation:

To determine whether a higher proportion of Italians than Americans prefer white champagne at weddings, we need to conduct a hypothesis test. First, we need to state the null hypothesis (H0) and the alternative hypothesis (Ha). In this case, H0: p1 = p2 (the proportion of Italians who prefer white champagne is equal to the proportion of Americans who prefer white champagne) and Ha: p1 > p2 (the proportion of Italians who prefer white champagne is greater than the proportion of Americans who prefer white champagne).

Next, we calculate the test statistic using the formula: z = (p1 - p2) / √(p*(1-p)*((1/n1) + (1/n2))), where p = (x1 + x2) / (n1 + n2), x1 and x2 are the number of Italians and Americans who prefer white champagne respectively, and n1 and n2 are the sample sizes of Italians and Americans respectively.

We then compare the test statistic to the critical value at a significance level of 0.05. If the test statistic is greater than the critical value, we reject the null hypothesis and conclude that a higher proportion of Italians than Americans prefer white champagne at weddings.

Answer 2

`z=\frac{0.247-0.15}{\sqrt{0.191(1-0.191)((1)/(300)+(1)/(400))}}=3.23`

`p_v =P(Z>3.23)=0.000619`

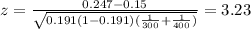
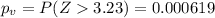
Comparing the p value with the significance level given
`\alpha=0.05` we see that
`p_v<\alpha` so we can conclude that we have enough evidence to reject the null hypothesis, and we can say that the proportion of Italians who prefer white champagne at​ weddings it's significantly higher than the proportion of Americans.

Step-by-step explanation:

1) Data given and notation

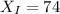`X_(I)=74` represent the number of Italians that preferred white​ champagne

`X_(A)=60` represent the number of Americans that preferred white​ champagne

`n_(I)=300` sample of Italians selected

`n_(A)=400` sample of Americans selected

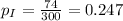`p_(I)=(74)/(300)=0.247` represent the proportion of Italians that preferred white​ champagne

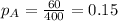`p_(A)=(60)/(400)=0.15` represent the proportion of Americans that preferred white​ champagne

z would represent the statistic (variable of interest)

`p_v` represent the value for the test (variable of interest)

`\alpha=0.05` significance level given

2) Concepts and formulas to use

We need to conduct a hypothesis in order to check if a higher proportion of Italians than Americans prefer white champagne at​ weddings, the system of hypothesis would be:

Null hypothesis:
`p_(I) - p_(A) \leq 0`

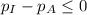
Alternative hypothesis:
`p_(I) - p_(A) > 0`

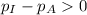
We need to apply a z test to compare proportions, and the statistic is given by:

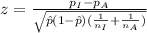`z=\frac{p_(I)-p_(A)}{\sqrt{\hat p (1-\hat p)((1)/(n_(I))+(1)/(n_(A)))}}` (1)

Where
`\hat p=(X_(I)+X_(A))/(n_(I)+n_(A))=(74+60)/(300+400)=0.191`

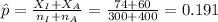
3) Calculate the statistic

Replacing in formula (1) the values obtained we got this:

`z=\frac{0.247-0.15}{\sqrt{0.191(1-0.191)((1)/(300)+(1)/(400))}}=3.23`

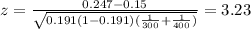
4) Statistical decision

Since is a right tailed test the p value would be:

`p_v =P(Z>3.23)=0.000619`

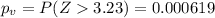
Comparing the p value with the significance level given
`\alpha=0.05` we see that
`p_v<\alpha` so we can conclude that we have enough evidence to reject the null hypothesis, and we can say that the proportion of Italians who prefer white champagne at​ weddings it's significantly higher than the proportion of Americans.