Answer:
1. 96.08%; 2. x=764.8; 3. 63.31%; 4. 93.57%; 5. 99.74%
Explanation:
The essential tool here is the standardized cumulative normal distribution which tell us, no matter the values normally distributed, the percentage of values below this z-score. The z values are also normally distributed and this permit us to calculate any probability related to a population normally distributed or follow a Gaussian Distribution. A z-score value is represented by:
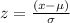 , and the density function is:
, and the density function is:
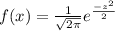
Where
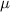 is the mean for the population, and
is the mean for the population, and
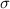 is the standard deviation for the population too.
is the standard deviation for the population too.
Tables for z scores are available in any Statistic book and can also be found on the Internet.
First Part
The combined math and verbal scores for students taking a national standardized examination for college admission, is normally distributed with a mean of 500 and a standard deviation of 170. If a college requires a minimum score of 800 for admission, what percentage of student do not satisfy that requirement?
For solve this, we know that
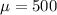 , and
, and
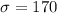 , so
, so
z =
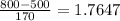 .
.
For this value of z, and having a Table of the Normal Distribution with two decimals, that is, the cumulative normal distribution for this value of z is F(z) = F(1.76) = 0.9608 or 96.08%. So, what percentage of students does not satisfy that requirement? The answer is 96.08%. In other words, only 3.92% satisfy that requirement.
Second Part
The combined math and verbal scores for students taking a national standardized examination for college admission, is normally distributed with a mean of 630 and a standard deviation of 200. If a college requires a student to be in the top 25 % of students taking this test, what is the minimum score that such a student can obtain and still qualify for admission at the college?
In this case
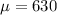 , and
, and
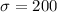 .
.
We are asked here for the percentile 75%. That is, for students having a score above this percentile. So, what is the value for z-score whose percentile is 75%? This value is z = 0.674 in the Standardized Normal Distribution, obtained from any Table of the Normal Distribution.
Well, having this information:
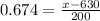 , then
, then
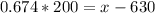
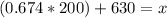

Then, the minimum score that a student can obtain and still qualify for admission at the college is x = 764.8. In other words, any score above it represents the top 25% of all the scores obtained and 'qualify for admission at the college'.
Third Part
[...] The collagen amount was found to be normally distributed with a mean of 69 and standard deviation of 5.9 grams per milliliter.
In this case
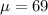 , and
, and
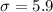 .
.
What percentage of compounds have an amount of collagen greater than 67 grams per milliliter?
z =
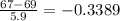 . The z-score tells us the distance from the mean of the population, then this value is below 0.3389 from the mean.
. The z-score tells us the distance from the mean of the population, then this value is below 0.3389 from the mean.
What is the value of the percentile for this z-score? That is, the percentage of data below this z.
We know that the Standard Distribution is symmetrical. Most of the tables give us only positive values for z. But, because of the symmetry of this distribution, z = 0.3389 is the distance of this value from the mean of the population. The F(z) for this value is 0.6331 (actually, the value for z = 0.34 in a Table of the Normal Distribution).
This value is 0.6331-0.5000=0.1331 (13.31%) above the mean. But, because of the symmetry of the Normal Distribution, z = -0.34, the value F(z) = 0.5000-0.1331=0.3669. That is, for z = -0.34, the value for F(z) = 36.69%.
Well, what percentage of compounds have an amount of collagen greater than 67 grams per milliliter?
Those values greater that 67 grams per milliliter is 1 - 0.3669 = 0.6331 or 63.31%.
What percentage of compounds have an amount of collagen less than 78 grams per milliliter?
In this case,
z =
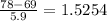 .
.
For this z-score, the value F(z) = 0.9357 or 93.57%. That is, below 78 grams per milliliter, the percentage of compounds that have an amount of collagen is 93.57%.
What exact percentage of compounds formed from the extract of this plant fall within 3 standard deviations of the mean?
We need here to take into account three standard deviations below the mean and three standard deviations above the mean. All the values between these two values are the exact percentage of compounds formed from the extract of this plant.
From the Table:
For z = 3, F(3) = 0.9987.
For z = -3, F(-3) = 1 - 0.9987 = 0.0013.
Then, the exact percentage of compounds formed from the extract of this plant fall within 3 standard deviations of the mean is:
F(3) - F(-3) = 0.9987 - 0.0013 = 0.9974 or 99.74%.