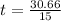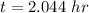Question

A small mirror is attached to a vertical wall, and it hangs a distance of 1.86 m above the floor. The mirror is facing due east, and a ray of sunlight strikes the mirror early in the morning and then again later in the morning. The incident and reflected rays lie in a plane that is perpendicular to both the wall and the floor. Early in the morning, the reflected ray strikes the floor at a distance of 3.80 m from the base of the wall. Later on in the morning, the ray is observed to strike the floor at a distance of 1.22 m from the wall. The earth rotates at a rate of 15.0˚ per hour. How much time (in hours) has elapsed between the two observations?

Answer 1

`t=2.044\ hr`

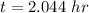
Step-by-step explanation:

From the schematic we can visualize the situation and the position of the rays falling on the floor.

Considering the given data from the lowest edge of the mirror.

• We get a triangle with height of 1.86 meters.

• In the first instance the base of the triangle is 3.8 meters.

• While in the second instance the base is 1.22 meters.
• Speed of rotation of earth,
  `\omega=15^(\circ)\ hr^(-1)`

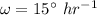
Now applying the trigonometric ratio to known sides in the first instance:

`tan\ \theta_1=(1.86)/(3.8)`

`\theta_1=26.08^(\circ)`

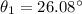
Applying the trigonometric ratio to known sides in the second instance:

`tan\ \theta_2=(1.86)/(1.22)`

`\theta_2=56.74^(\circ)`

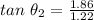
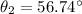
Now by the law of reflection we know that the angle of incidence is equal to the angle of reflection. So the sun would have been at the same angle on the opposite side of the normal.

Hence the change in angle of the sun with respect to the mirror (also the earth)

`\Delta \theta=\theta_2-\theta_1`

`\Delta \theta=56.74-26.08`

`\Delta \theta=30.66^(\circ)`

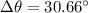
Now the time past for this change:

`t=(\Delta \theta)/(\omega)`

`t=(30.66)/(15)`

`t=2.044\ hr`