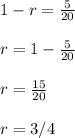Question

Please... ?

The first term of an infinite geometric progression is 5 and the sum of its terms is 20. What is the common ratio of the progression?

Answer 1

• The common ratio of the progression is 3/4

Step-by-step explanation:

A geometric progression is a sequence of terms in which the consecutive terms have a constant ratio; thus, each term is equal to the previous one multiplied by a constant value:

`First\ term=a_1\\\\ Second\ term=a_2=a_1* r\\\\ Third\ term=a_3=a_2* r=a_1* r^2\\\\n_(th)\ term=a_n=a_(n-1)* r=a_1* r^(n-1)`

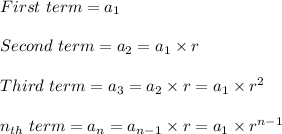
A infinite geometric progression may have a finite sum. When the absolute value of the ratio is less than 1, the sum of the infinite geometric progression has a finite value equal to:

• 
  `S_(\infty)=(a_1)/(1-r)`

Thus, the information given translates to:

`a_1=5\\ \\ S_(\infty)=20=(5)/(1-r)`

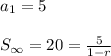
Now you can solve for the constant ratio, r:

`1-r=(5)/(20)\\ \\ r=1-(5)/(20)\\ \\ r=(15)/(20)\\  \\ r=3/4`