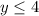Question

5 What is the range of y = -x2 - 2x + 3?
Ax<4
B x2-4
c y<4
Dy2-4

Answer 1

`y\leq 4`

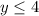
Explanation:

we have

`y=-x^(2)-2x+3`

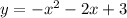
This is a vertical parabola open downward (the leading coefficient is negative)

The vertex is a maximum

The vertex is the point (h,k)

The range of the function is the interval (-∞,k]

so

`y\leq k`

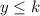
Convert the quadratic equation in vertex form

Factor -1 leading coefficient

`y=-(x^(2)+2x)+3`

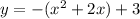
Complete the square

`y=-(x^(2)+2x+1)+3+1`

`y=-(x^(2)+2x+1)+4`

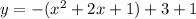
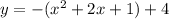
Rewrite as perfect squares

`y=-(x+1)^(2)+4`

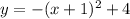
The vertex is the point (-1,4)

therefore

The range is
`y\leq 4`