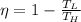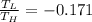Answer:
So the ratio will be
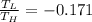
Step-by-step explanation:
We have given heat engine absorbs 450 joule from high temperature reservoir
So
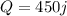
As the heat engine expels 290 j
So work done W = 290 J
We know that efficiency
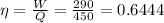
It is given that efficiency of the engine only 55 % of Carnot engine
So efficiency of Carnot engine

Efficiency of Carnot engine is