Answer:
tex]\lambda_{Be}[/tex] = 22.78 nm
Step-by-step explanation:
Bohr's model for the hydrogen atom has been used by other atoms with a single electric charge by changing the number of charges by the charge of the new atom (atomic number)
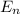 = k e² / 2a₀ (1 /n²)
= k e² / 2a₀ (1 /n²)
ao = h'² / k m e² h' = h/2πi
For another atom with a single electron in the last layer
a₀ ’= h’² / k m (Ze)²
a₀ ’= a₀ / Z²
Therefore, when replacing in the equation
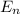 = - Z² Eo/n²
= - Z² Eo/n²
E₀ = 13,606 eV
The transition occurs when the electron stops from one level to another
 -
-
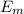 = Z² E₀ (1 / n² - 1 / m²) = Z² ΔE
= Z² E₀ (1 / n² - 1 / m²) = Z² ΔE
Let's relate this expression to the wavelength
c = λ f
E = h f
E = h c /λ
h c / λ = Z² ΔE
λ = 1 / Z² (hc / ΔE)
λ = 1 / Z² λ_hydrogen
Let's apply this last equation to our case
Lithium Z = 3
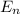 = - 9 Eo / n²
= - 9 Eo / n²
40.5 10-9 = 1/9 λ_hydrogen
Beryllium Z = 4
λ = 1/16 λ_hydrogen
Let's write our two equations is and solve
40.5 10-9 = 1/9 λ_hydrogen
tex]\lambda_{Be}[/tex] = 1/ 16 λ_hydrogen
40.5 10⁻⁹ = 1/9 (16
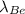 )
)
tex]\lambda_{Be}[/tex] = 40.5 9/16
tex]\lambda_{Be}[/tex] = 22.78 nm