Answer:
His conclusion was incorrect.
Explanation:
Since, slope of a line passes through
 and
and
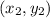 is,
is,

Thus, the slope between (-1, 4) and (0, 0),
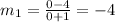
Also, the slope between (2, 7) and (3, 3),
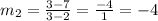
Now,
There are two cases possible,
Case 1 : line passes through (-1, 4) and (0, 0) is parallel to line passes through (2, 7) and (3, 3)
Case 2 : (-1, 4), (0, 0), (2, 7) and (3, 3) are on the same line
Hence, we can not say that they are on same line only after getting same slope.
i.e. his conclusion was incorrect.