Answer:
The equation has no solutions .
Explanation:
Given as :
The linear equation is
 (24 -16 x) =
(24 -16 x) =
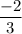 (18 x -27)
(18 x -27)
Or,
 ×24 -
×24 -
 × 16 x =
× 16 x =
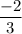 × 18 x -
× 18 x -
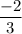 × 27
× 27
Or,
 ×24 -
×24 -
 × 16 x =
× 16 x =
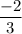 × 18 x +
× 18 x +
 × 27
× 27
Or,
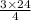 -
-
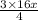 =
=
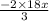 +
+
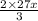
Or, 3 × 6 - 3 × 4 x = - 2 × 6 x + 2 × 9
Or, 18 - 12 x = - 12 x + 18
Or, 18 - 18 = - 12 x + 12 x
or, 0 = 0
∵ The linear equation has no variables , so the equation has no solution
Hence,The equation has no solutions . Answer