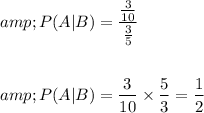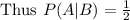According to the general equation for conditional probability, if P(ANB) = 3/10 and P(B)= 3/5, then P(A I B) is

Solution:
Given that, According to the general equation for conditional probability,
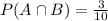
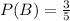
We need to find

The required formula is:
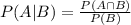
Substituting the values,