Question

Hard times In June 2010, a random poll of 800 working men found that 9% had taken on a second job to help pay the bills. (www.careerbuilder) a) Estimate the true percentage of men that are taking on second jobs by constructing a 95% confidence interval. b) A pundit on a TV news show claimed that only 6% of work-ing men had a second job. Use your confidence interval to test whether his claim is plausible given the poll data.

Answer 1

a) The 95% confidence interval would be given (0.0702;0.1098).

We can conclude that the true population proportion at 95% of confidence is between (0.0702;0.1098)

b) Since the confidence interval NOT contains the value 0.06 we have anough evidence to reject the claim at 5% of significance.

Explanation:

Previous concepts

A confidence interval is "a range of values that’s likely to include a population value with a certain degree of confidence. It is often expressed a % whereby a population means lies between an upper and lower interval".

The margin of error is the range of values below and above the sample statistic in a confidence interval.

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

`p` represent the real population proportion of interest

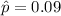`\hat p =0.09` represent the estimated proportion for the sample

n=800 is the sample size required

`z` represent the critical value for the margin of error

Confidence =0.95 or 95%

Part a

The population proportion have the following distribution

`p \sim N(p,\sqrt{(p(1-p))/(n)})`

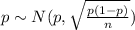
The confidence interval would be given by this formula

`\hat p \pm z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

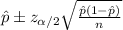
For the 95% confidence interval the value of
`\alpha=1-0.95=0.05` and
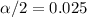`\alpha/2=0.025`, with that value we can find the quantile required for the interval in the normal standard distribution.

`z_(\alpha/2)=1.96`

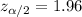
The margin of error is given by :

`Me=z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

`Me=1.96 \sqrt{(0.09(1-0.09))/(800)}=0.0198`

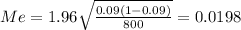
And replacing into the confidence interval formula we got:

`0.09 - 1.96 \sqrt{(0.09(1-0.09))/(800)}=0.0702`

`0.09 + 1.96 \sqrt{(0.108(1-0.09))/(800)}=0.1098`

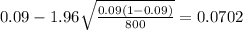
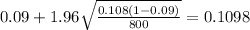
And the 95% confidence interval would be given (0.0702;0.1098).

We can conclude that the true population proportion at 95% of confidence is between (0.0702;0.1098)

Part b

Since the confidence interval NOT contains the value 0.06 we have anough evidence to reject the claim at 5% of significance.