Answer: 0.0055
Explanation:
Let
 denotes the sample mean amount that can has.
denotes the sample mean amount that can has.
We assume that cans of Coke are filled so that the actual amounts are normally distributed with a mean of 12.00 oz and a standard deviation of 0.1 oz.
i.e.
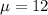 and
and

sample size : n= 8
Then, the probability that a sample of 88 cans will have a mean amount of at least 12.09:
![P(\overline{x}\geq12.09)=1-P(\overline{x}<12.09)\\\\=1-P(\frac{\overline{x}-\mu}{(\sigma)/(√(n))}<(12.09-12)/((0.1)/(√(8))))\\\\=1-P(z<2.5456)\ \ [\because z=\frac{\overline{x}-\mu}{(\sigma)/(√(n))}]\\\\=1-0.9945\ \ [\text{By z-table}]\\\\=0.0055](https://img.qammunity.org/2020/formulas/mathematics/high-school/r4i5f9iic0vusyad0q7g9mx29139ppckkq.png)
Hence, the required sample size = 0.0055