Answer:
A) The selling price for each necklaces is $8.625
B) The profit mark in each necklaces is 15%
Explanation:
Given as :
The total cost of two necklaces = c.p = $15
The markup percentage for the necklaces = m = 15%
Let The selling price for both necklaces = s.p
A ) Now, From markup method
m% =
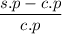
or, 15% =
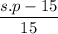
or, 15% =
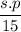 - 1
- 1
or,
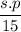 = 1 + 15%
= 1 + 15%
or,
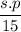 = 1 +
= 1 +
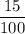
or,
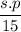 =
=
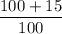
or,
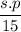 =
=
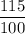
∴ s.p =

I.e s.p = $17.25
So, selling price of two necklaces = s.p = $17.25
or, selling price of one necklaces = s.p =
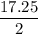 = $8.625
= $8.625
Hence, The selling price for each necklaces is $8.625
Now, Again
B) ∵ The total cost of two necklaces = c.p = $15
So, The cost price of one necklaces =
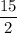 = $7.5
= $7.5
∴ profit% for each necklace =
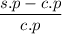
i.e profit% for each necklace =
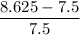
Or, profit% for each necklace =

Or, profit% for each necklace = 0.15
So, The profit make in each necklaces = 15%
Hence, The profit mark in each necklaces is 15%
Answer