Question

A ball is thrown vertically upward from the top of a building with an initial velocity of 96 feet per second. The ball's h height after t seconds is given by the equation h = −16t2 + 96t + 100. How long will it take for the ball to hit the ground? (to the nearest tenth of a second)

A) 5.9 seconds
B) 6.3 seconds
C) 6.9 seconds
D) 7.2 seconds

Answer 1

Option C

6.9 seconds

Explanation:

When the ball hits the ground, h=0 therefore

-16t^{2}+96t+100=0

Solving the quadratic equation using quadratic formula where

`x=\frac {-b\bar +\sqrt{b^(2)-4ac}}{2a}`

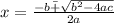
Substituting -16 for a, 96 for b and 100 for c then

`x=\frac {-96\bar +\sqrt{96^(2)-(4* -16* 100)}}{2* -16}`

`x=6.960498311\approx 6.9\\-0.960498311\approx -1`

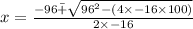
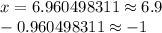
Since time can't be negative, therefore, t=6.9