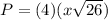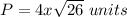Question

The lengths of the diagonals of a rhombus are 2x and 10x. What expression gives the perimeter of the rhombus?

A. 42
B.
196

Answer 1

`P=4x√(26)\ units`

Explanation:

we know that

The sides of a rhombus are all congruent and the diagonals are perpendicular bisectors of each other

so

Applying the Pythagorean Theorem

`c^2=a^2+b^2`

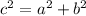
where

c is the length side of the rhombus

a and b are the semi-diagonals

we have

`a=2x/2=x\ units\\b=10x/2=5x\ units`

substitute the values

`c^2=x^2+(5x)^2`

`c^2=26x^2`

`c=x√(26)\ units`

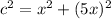
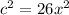
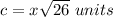
To find out the perimeter of the rhombus multiply the length side by 4

`P=(4)(x√(26))`

`P=4x√(26)\ units`