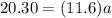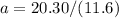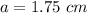Question

The area of the regular octagon is 10.15 cm2.

A regular octagon has sides with lengths of 1.45 centimeters.

What is the measure of the apothem, rounded to the nearest hundredth of a centimeter?

Answer 1

B) 1.75 cm

~Congrats you're on the Cumulative Exam, good job!

Answer 2

The measure of the apothem is 1.75 cm

Explanation:

we know that

The area of a regular polygon is equal to

`A=(1)/(2)Pa`

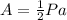
where

P is the perimeter of the regular polygon

a is the apothem of the regular polygon

Find the perimeter P

The perimeter of the regular octagon is equal to the length side of the octagon multiplied by 8 (the number of sides)

so

`P=1.45(8)=11.6\ cm`

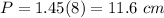
Find the apothem

we have

`P=11.6\ cm`

`A=10.15\ cm^2`

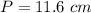
substitute in the formula

`A=(1)/(2)Pa`

`10.15=(1)/(2)(11.6)a`

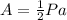
solve for a

`20.30=(11.6)a`

`a=20.30/(11.6)`

`a=1.75\ cm`