Option B
Surface area of cylinder is 24π square centimeter
Solution:
Given that volume of a cylinder is
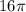 cubic centimeter
cubic centimeter
Radius = 2 cm
To find: surface area of cylinder
The volume of cylinder is given as:
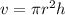
Where "r" is the radius of cylinder and "h" is the height of cylinder
Substituting v = 16π and r = 2 in above formula,
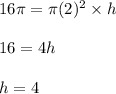
Thus height of cylinder is 4 cm
The surface area of cylinder is given as:
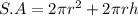
Substituting h = 4 and r = 2 in above formula,
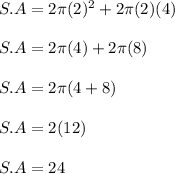
Thus surface area of cylinder is 24π square centimeter